Unlock trigonometry’s power! Master complex trigonometric problems effortlessly with this comprehensive guide and identities cheat sheet. From basic definitions to advanced applications, this resource provides everything you need to simplify expressions, solve equations, and prove trigonometric relationships with confidence. Download the convenient PDF cheat sheet to have essential trig identities at your fingertips, anytime, anywhere. Go beyond memorization and understand the derivations and applications of each identity for a deeper grasp of trigonometric concepts.
Trig Fundamentals: A Quick Refresher
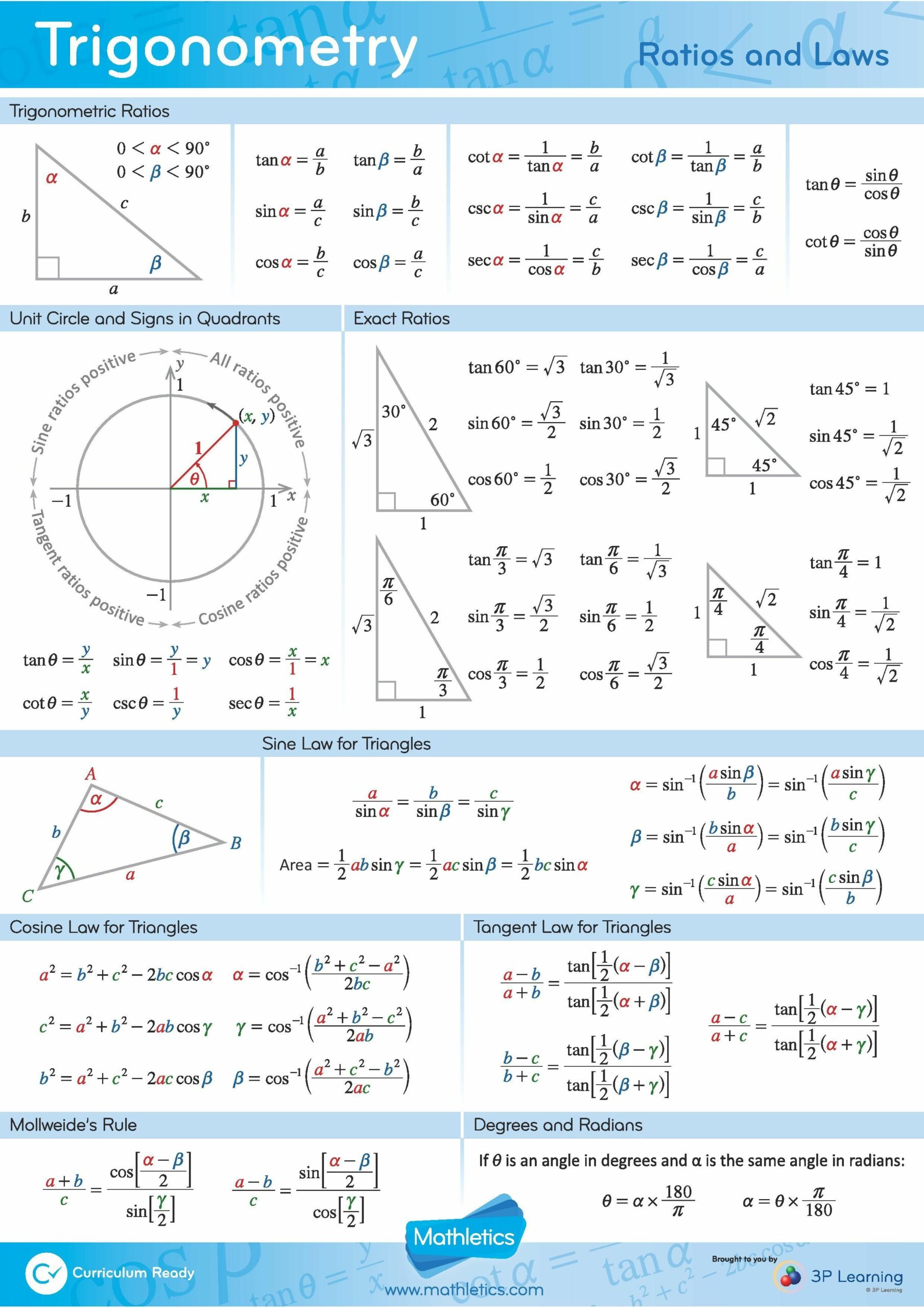
Before diving into the identities, let’s review the basics. Trigonometric functions (sine, cosine, tangent, cosecant, secant, and cotangent) describe relationships within right triangles and on the unit circle. They essentially explain how angles and sides relate.
Visualizing these functions on the unit circle—a circle with radius 1—can be helpful. Imagine a right triangle inside, with its hypotenuse along the circle’s edge. The trigonometric functions correspond to ratios of the triangle’s sides. This visual clarifies how identities work.
Essential Trig Identities: Your Handy Toolkit
A trigonometric identities cheat sheet is your secret weapon for conquering the world of angles and triangles. It’s a curated list of essential formulas, organized by category for easy navigation. This comprehensive guide clarifies the connections between sine, cosine, tangent, and their counterparts.
Here’s a handy table summarizing these essential identities:
| Category | Identity | What it likely suggests |
|---|---|---|
| Pythagorean | sin²θ + cos²θ = 1 1 + tan²θ = sec²θ 1 + cot²θ = csc²θ | These identities, derived from the Pythagorean theorem, show the relationships between sine & cosine, tangent & secant, and cotangent & cosecant on the unit circle. |
| Reciprocal | csc θ = 1/sin θ sec θ = 1/cos θ cot θ = 1/tan θ | These demonstrate inverse relationships. Each function is the reciprocal of another. |
| Quotient | tan θ = sin θ / cos θ cot θ = cos θ / sin θ | Tangent and cotangent are defined as ratios of sine and cosine. |
| Co-function | sin(π/2 – θ) = cos θ cos(π/2 – θ) = sin θ | Sine and cosine are complementary; the sine of an angle is the cosine of its complement, and vice-versa. This applies to other co-function pairs. |
| Sum & Difference | sin(α ± β) = sinαcosβ ± cosαsinβ cos(α ± β) = cosαcosβ ∓ sinαsinβ tan(α ± β) = (tanα ± tanβ) / (1 ∓ tanαtanβ) | These formulas expand trigonometric functions of the sum or difference of two angles. |
| Double-Angle | sin(2θ) = 2sinθcosθ cos(2θ) = cos²θ – sin²θ = 2cos²θ – 1 = 1 – 2sin²θ tan(2θ) = 2tanθ / (1 – tan²θ) | These are special cases of sum formulas (α = β), providing concise expressions for multiples of an angle. Note multiple forms for cos(2θ). |
| Half-Angle | sin(θ/2) = ±√[(1 – cosθ)/2] cos(θ/2) = ±√[(1 + cosθ)/2] tan(θ/2) = ±√[(1 – cosθ)/(1 + cosθ)] | These formulas express trigonometric functions of half an angle. The ± sign depends on the quadrant of θ/2. |
Applying Trig Identities: Practical Examples
Let’s move beyond theory. Suppose you encounter the expression sin(x)tan(x) + cos(x). Using the quotient identity (tan(x) = sin(x)/cos(x)), substitution and simplification reveal the result: sec(x). This demonstrates how identities make complex problems manageable. More complex examples would follow a similar process of identification, substitution, and simplification.
Periodicity: The Cyclical Nature of Trig Functions
Trigonometric functions are periodic, meaning their values repeat in a predictable cycle. Sine and cosine repeat every 2π radians (360°), while tangent repeats every π radians (180°). This is visually represented by the wave-like graphs of these functions. Periodicity is crucial for understanding that trigonometric equations can have infinite solutions.
Common Pitfalls to Avoid: Navigating Trig Traps
Even with a cheat sheet (trig identity sheet), mistakes happen. Common errors include mixing up signs in sum and difference formulas and forgetting the ± in half-angle formulas. Meticulousness is key; double-check your work.
Beyond the Basics: Untapped Potential
While this cheat sheet covers essential identities, current research continues to explore trigonometric applications in diverse fields like physics, engineering, computer graphics, and music theory. This knowledge is likely the tip of the iceberg. Future research may reveal further nuances and connections, highlighting the dynamic nature of mathematics.
This cheat sheet is more than just a list of formulas; it’s your compass in the world of trigonometry. Use it wisely, practice consistently, and watch your understanding of this fascinating field flourish. Don’t just conquer trig – own it. Explore further with interactive quizzes and practice problems (coming soon!) to solidify your understanding. A printable PDF version is also in development for on-the-go access.
- China II Review: Delicious Food & Speedy Service - April 17, 2025
- Understand Virginia’s Flag: History & Debate - April 17, 2025
- Explore Long Island’s Map: Unique Regions & Insights - April 17, 2025