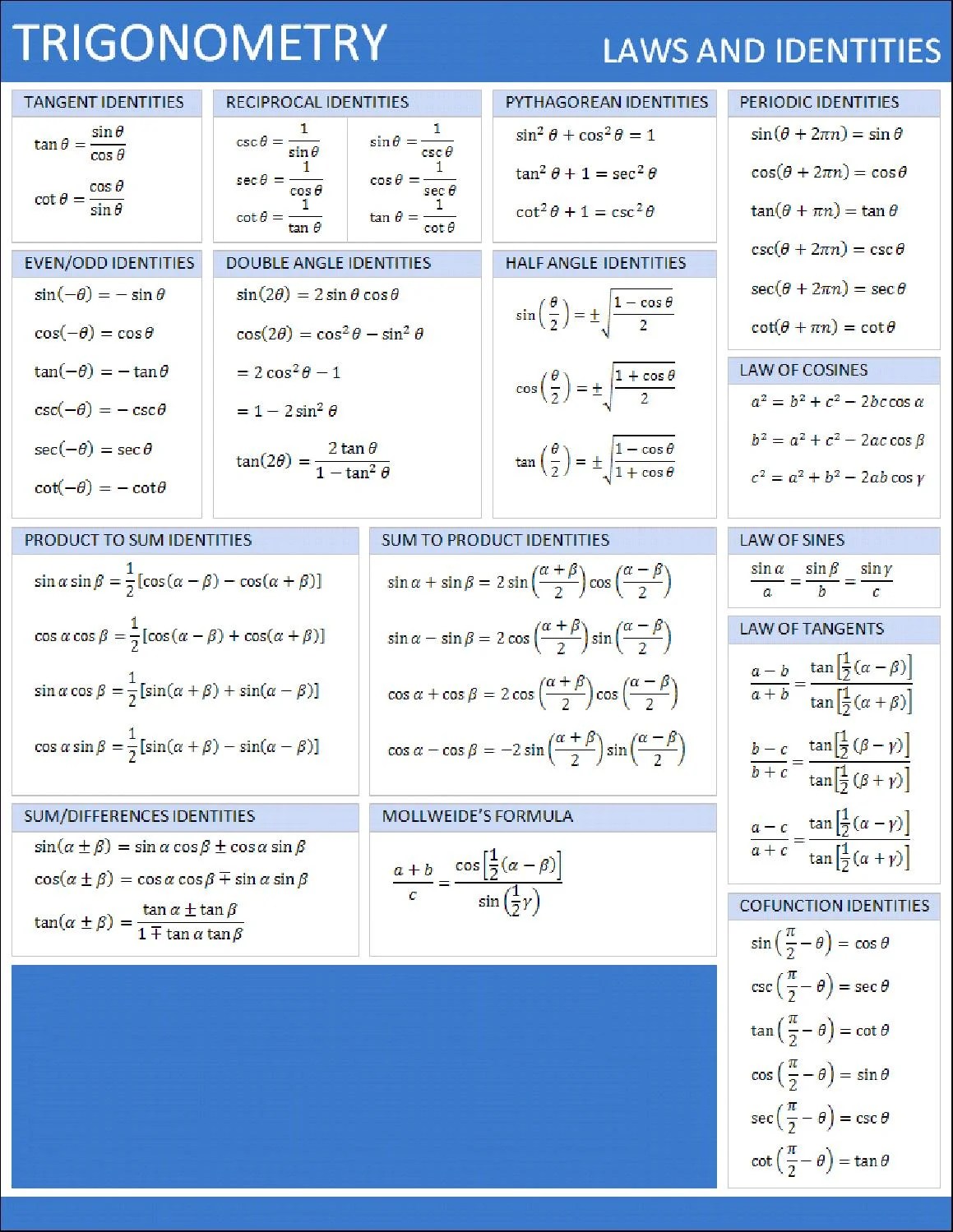
Trig identities. They’re the fundamental building blocks of trigonometry, the keys to unlocking complex equations, and often, the source of many a student’s frustration. But fear not! This comprehensive guide will transform you from trig-timid to trig-triumphant. We’ll break down the essential identities, explore their connections, and equip you with the ultimate trig identities cheat sheet—complete with printable PDFs [https://www.lolaapp.com/trig-identities-sheet]—to conquer any trigonometric challenge.
Essential Trig Identities: Your Foundational Toolkit
Trigonometric identities are equations that hold true for all values of the involved variables. They’re your go-to tools for simplifying expressions, verifying equations, and proving trigonometric relationships. Let’s start with the bedrock:
Reciprocal Identities: Flipping the Script
These identities define the reciprocal relationships between trigonometric functions. Think of them as the flip side of the coin:
csc θ = 1/sin θ
sec θ = 1/cos θ
cot θ = 1/tan θ
These simple yet powerful relationships can often make a complicated expression much more manageable. For example, if you see 1/sin θ in an equation, you can instantly swap it for csc θ.
Pythagorean Identities: The Triangle’s Theorem
Just like the Pythagorean theorem (a² + b² = c²) governs right triangles, Pythagorean identities rule the world of trigonometry. These identities are derived from the unit circle and reveal the inherent relationships between sine, cosine, tangent, secant, cosecant, and cotangent:
sin²θ + cos²θ = 1 (This one’s a classic!)
1 + tan²θ = sec²θ
1 + cot²θ = csc²θ
These are invaluable for simplifying expressions and proving trigonometric relationships, allowing you to manipulate equations and reveal hidden connections.
Quotient Identities: Bridging the Gap
These identities connect tangent and cotangent to sine and cosine, establishing a crucial link between these fundamental functions:
tan θ = sin θ / cos θ
cot θ = cos θ / sin θ
This allows you to express tangent and cotangent in terms of sine and cosine, offering flexibility in how you approach a problem. For instance, if you have an equation with tangent and you prefer to work solely with sines and cosines, these identities make the conversion straightforward.
Cofunction Identities: Complementary Connections
Cofunction identities illuminate the relationships between trigonometric functions of complementary angles (angles that sum to 90° or π/2 radians):
sin(π/2 - θ) = cos θ
cos(π/2 - θ) = sin θ
tan(π/2 - θ) = cot θ
These identities can simplify expressions with complementary angles, offering a shortcut to simplify and solve.
Advanced Trig Identities: Expanding Your Arsenal
Once you’ve mastered the foundational identities, you’re ready to explore more advanced tools:
Double-Angle Identities: Doubling Down
These identities express trigonometric functions of double angles (2θ) in terms of single angles (θ):
sin(2θ) = 2sinθcosθ
cos(2θ) = cos²θ - sin²θ = 2cos²θ - 1 = 1 - 2sin²θ (Note that cosine has three equivalent forms).
tan(2θ) = (2tanθ) / (1 - tan²θ)
These formulas are essential for dealing with situations where angles are doubled, allowing you to rewrite expressions in a more workable format.
Sum and Difference Identities: Adding and Subtracting Angles
These identities deal with trigonometric functions of the sum or difference of two angles (α and β):
These identities are especially valuable when working with combinations of angles, providing a way to decompose complex expressions into simpler parts.
Half-Angle Identities: Halving the Angle
These identities express trigonometric functions of half angles (θ/2) in terms of the full angle (θ):
sin(θ/2) = ±√[(1 - cosθ)/2] (Don’t forget the ±; it depends on the quadrant of θ/2)
A cheat sheet is a powerful tool, but it’s most effective when combined with understanding and practice:
Practice Makes Perfect: Regular practice is crucial. Use your cheat sheet as a guide, but challenge yourself to recall identities from memory.
Look for Patterns: Notice the symmetries and relationships between different identities. This can help you remember them more easily.
Start with the Complex Side: When proving identities, it’s often helpful to start with the more complex side and try to simplify it.
Convert to Sine and Cosine: Rewriting everything in terms of sine and cosine can often simplify the problem.
Factor and Use Conjugates: Algebraic techniques like factoring and using conjugates can also be useful in simplifying expressions.
Be Mindful of the ±: Pay close attention to the ± signs in half-angle formulas, as they depend on the quadrant of the angle.
Trig Identities in the Real World: Beyond the Classroom
While they may seem abstract, trig identities have real-world applications. They’re used in fields like physics (modeling wave motion and projectile motion), music production (analyzing sound waves), engineering (calculating forces and designing structures), and architecture (determining angles and lengths in building design). Having a strong grasp of these identities opens doors to understanding and applying these concepts in diverse fields.
Why a Trig Identities Cheat Sheet? Your Key to Success
A trig identities cheat sheet provides a readily available, organized summary of these essential formulas. This quick reference is invaluable for problem-solving, minimizing errors, and saving valuable time. But it’s more than just a list of formulas. A well-designed cheat sheet, like the one provided [https://www.lolaapp.com/trig-identities-sheet], can also be a powerful learning tool. By actively engaging with the cheat sheet—testing yourself, looking for patterns, and understanding the relationships between identities—you can move beyond rote memorization and develop a deeper understanding of trigonometric concepts. This comprehensive approach, combining a well-structured cheat sheet with active learning strategies, is your key to unlocking true trigonometric mastery.
This comprehensive guide provides you with a robust foundation in trig identities and a handy quick-reference sheet. Now, go forth and conquer those trig challenges!
Hello, My love for writing is rivaled only by my passion for in-depth research. While many may skim the surface, I dive deep, seeking the hidden gems of information that give a story texture and depth. Every word I write is backed by hours of exploration, ensuring that my work is not just compelling, but also meticulously accurate. Join me on a journey where every piece is a deep dive into the realms of knowledge and storytelling.
