Unlock the power of quadratic factoring with this comprehensive guide. Whether you’re a student gearing up for exams or an educator seeking top-notch resources, you’ll find everything you need to become a quadratic factoring expert. Let’s embark on this mathematical journey together!
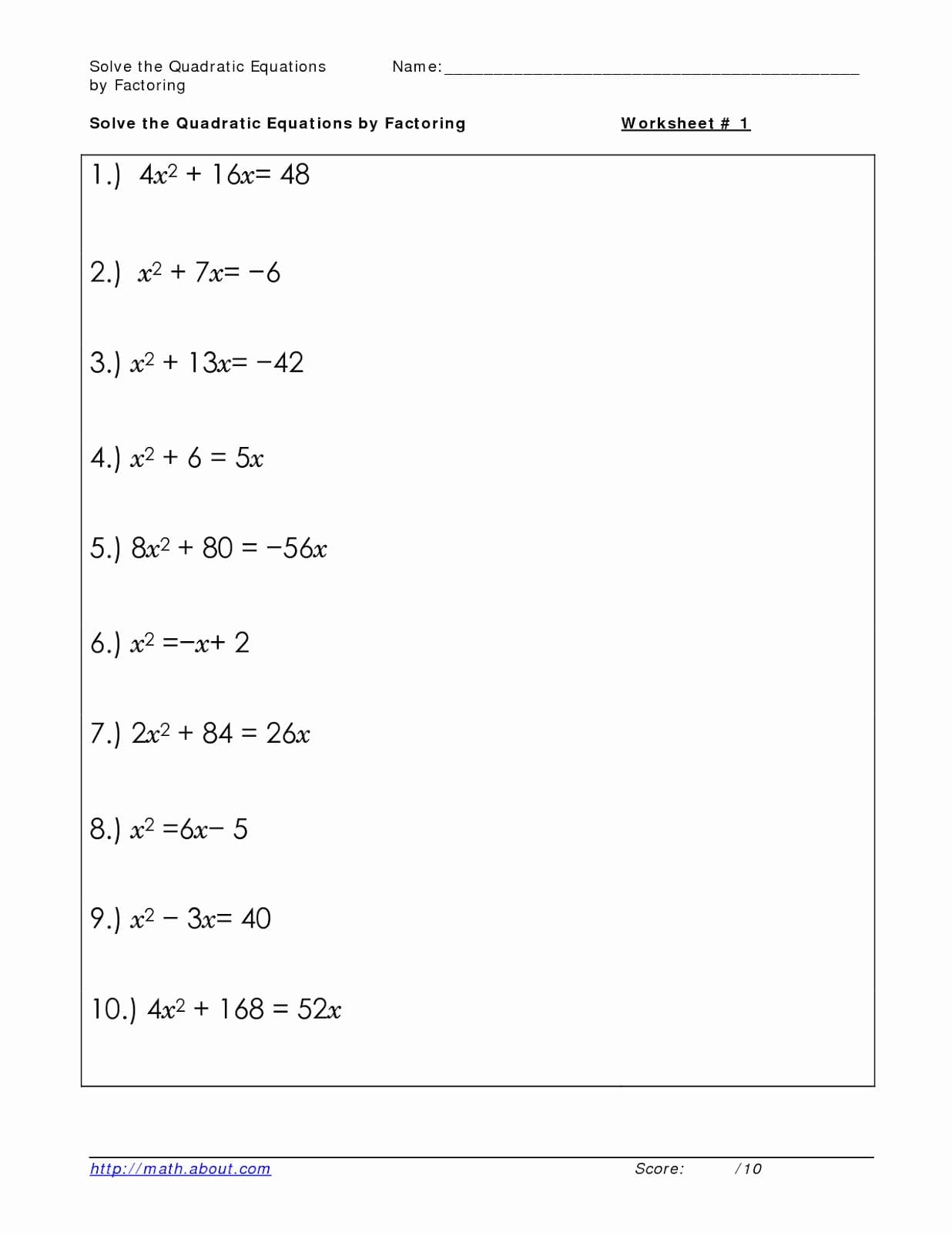
Ready to conquer quadratic equations? This guide, complete with free factoring quadratics worksheets, provides the tools and techniques you need to succeed. Factoring, essentially reverse multiplication, is a cornerstone of algebra, crucial for solving quadratic equations prevalent in fields from physics to finance. Think of it as discovering the pieces that, when multiplied, create the quadratic expression.
What’s Inside Our Worksheets?
Our worksheets are designed for progressive learning, starting with the basics and gradually increasing in complexity. They cover various methods, including “splitting the middle term” and the quadratic formula.
Conceptual Clarity: We explain the why behind each method, not just the how.
Step-by-Step Examples: Numerous examples demonstrate each factoring technique.
Incremental Progression: Start simple, then tackle more challenging problems.
Real-World Connections: See how factoring applies to real-world scenarios.
Free and Accessible: Printable PDFs and interactive online tools.
Different Worksheet Types
We offer diverse worksheets to target specific skills:
Basic Trinomials (a=1): Focuses on factoring expressions like x² + bx + c.
Complex Expressions (a>1): Factoring ax² + bx + c, where ‘a’ is greater than 1.
Tricky Quadratics: Intricate quadratics with multiple variables, fractions, or radicals.
Solving Quadratic Equations: Practice finding roots using factoring.
Quadratic Form: Factoring expressions resembling quadratics, possibly with higher powers.
Worksheet Type
Description
Difficulty Level
Basic Trinomials (a=1)
Factoring x² + bx + c
Beginner
Complex Expressions (a>1)
Factoring ax² + bx + c
Intermediate
Tricky Quadratics
Factoring expressions with multiple variables, fractions, or radicals
Learn from Mistakes: Our resources highlight common errors to avoid.
Explore Real-World Applications: Understanding how factoring is used in various fields makes learning more engaging.
With practice and our resources, you can conquer quadratic factoring. Remember, it’s a marathon, not a sprint. Take your time, practice consistently, and don’t hesitate to seek help. If you’re looking to boost reading skills, check out our CVCE words. Need practice with time? Try our elapsed time worksheets.
Unlocking Quadratic Equations: The Definitive Guide to Factoring
Mastering quadratic factoring is akin to unlocking a mathematical code, breaking down complex expressions into simpler parts. This essential skill allows you to solve equations, interpret graphs, and access more advanced math concepts. It’s building a solid foundation for future mathematical success.
What Does “Mastering” Mean?
Mastery doesn’t require wizardry, just comfort with various quadratic expressions and selecting the right factoring method. This mathematical intuition develops with practice, just like any other skill.
Essential Factoring Techniques
Greatest Common Factor (GCF): Identifying the largest term that divides evenly into all parts of the expression.
Factoring Trinomials (a=1): Finding two numbers that multiply to ‘c’ and add up to ‘b’ in expressions like x² + bx + c.
Factoring Trinomials (a>1): Utilizing methods like the “AC method” or grouping for expressions like 2x² + 7x + 3. Trial and error may also be useful.
Difference of Squares: Recognizing patterns like x² – 9 and applying the formula (a² – b²) = (a + b)(a – b).
Perfect Square Trinomials: Identifying patterns like x² + 6x + 9 and using formulas like (a² + 2ab + b²) = (a + b)².
Tips for Factoring Success
Double-Check Your Work: Multiply factors to verify correctness.
Choose the Right Tool: Select the appropriate method for each expression.
Practice Regularly: Hone your skills with consistent practice using free online worksheets.
Seek Assistance: Don’t hesitate to ask for help when needed.
Mastering quadratic factoring extends beyond tests. It’s a fundamental skill with applications in physics, engineering, and computer science. It’s about developing problem-solving abilities applicable in various contexts. Remember, mathematics is constantly evolving, and new research may lead to different factoring approaches in the future.
Unlocking Quadratic Equations: Why Factoring Worksheets are Key to Success
We’ve established factoring’s importance, but why are worksheets so vital? They provide the “practice court” for honing your skills and building confidence.
The Power of Repetition
Worksheets offer structured practice, reinforcing concepts and building mental pathways for automatic factoring. Each problem solved solidifies understanding, like becoming fluent in a new language.
Real-World Relevance
Factoring has practical applications beyond textbooks. From bridge design to stock market predictions, quadratics appear in surprising places. Factoring skills unlock solutions to real-world problems.
Personalized Learning
Worksheets come in various forms, catering to different skill levels and learning styles. They offer personalized “training” tailored to your specific needs.
Building a Strong Foundation
Mastering factoring lays a foundation for advanced math concepts like calculus, differential equations, and linear algebra. It’s an essential building block.
Boosting Problem-Solving Skills
Factoring requires strategic thinking, trying different approaches, and creativity. Worksheets help develop critical problem-solving skills valuable beyond math.
Mathematical research is ongoing. New techniques and perspectives are constantly emerging. Perhaps more efficient factoring approaches await discovery. Keep exploring and practicing!
Conquer Quadratic Factoring: How Worksheets Unlock Mastery (Step-by-Step)
Ready to conquer quadratic factoring? Worksheets are your secret weapon, offering focused practice for mastery. They are carefully designed to build skills incrementally, pinpoint strengths and weaknesses, and provide immediate feedback through answer keys.
Using Worksheets Effectively
Step 1: Lay the Foundation: Start with worksheets covering GCF and difference of squares. Step 2: Level Up: Progress to more challenging worksheets with trinomials. Step 3: Dig Deeper: Understand the why behind each technique, possibly exploring different approaches like the “AC method” or “guess and check.” Step 4: Learn from Missteps: Review incorrect answers to identify areas needing more attention. Step 5: Seek Help When Needed: Don’t hesitate to ask teachers, tutors, or consult online resources.
Common Factoring Techniques
Technique
Example
Factored Form
GCF
2x² + 4x
2x(x + 2)
Difference of Squares
x² – 9
(x – 3)(x + 3)
Trinomial (leading coefficient 1)
x² + 5x + 6
(x + 2)(x + 3)
Trinomial (leading coefficient other than 1)
2x² + 7x + 3
(2x + 1)(x + 3)
Grouping
x³ + 2x² + 3x + 6
(x² + 3)(x + 2)
Remember, mathematics is a dynamic field. While these techniques are currently standard, ongoing research may lead to new approaches. Consistent practice and focused understanding will lead you to mastery. Worksheets are your reliable companions, providing support and guidance. Take your time, work thoughtfully, and celebrate your progress!
Hello, My love for writing is rivaled only by my passion for in-depth research. While many may skim the surface, I dive deep, seeking the hidden gems of information that give a story texture and depth. Every word I write is backed by hours of exploration, ensuring that my work is not just compelling, but also meticulously accurate. Join me on a journey where every piece is a deep dive into the realms of knowledge and storytelling.
