Imagine pushing a child on a swing. You give them a good shove, and they swing back and forth. But the swinging eventually slows and stops due to damping. The damping ratio measures how quickly this settling down occurs. It’s a dimensionless number, usually between zero and one, that describes a system’s tendency to oscillate after a disturbance. It’s a crucial concept in various fields, from earthquake engineering to designing smooth car rides. Discover the hidden treasures at the crater of diamonds, where you can dig for your own diamonds, and imagine the damping ratio at play as your tools vibrate against the earth.
Different Damping Behaviors: Underdamped, Critically Damped, and Overdamped
Think of the swing example. A frictionless swing (zero damping) would swing forever. A swing dipped in molasses (high damping) would slowly return to rest without oscillating. Most real-world systems fall somewhere in between: they oscillate, but each swing is smaller than the last (underdamped). The damping ratio quantifies how quickly these oscillations decay. Critically damped systems return to equilibrium as fast as possible without overshooting.
Calculating Damping Ratio: The Formula and Its Components
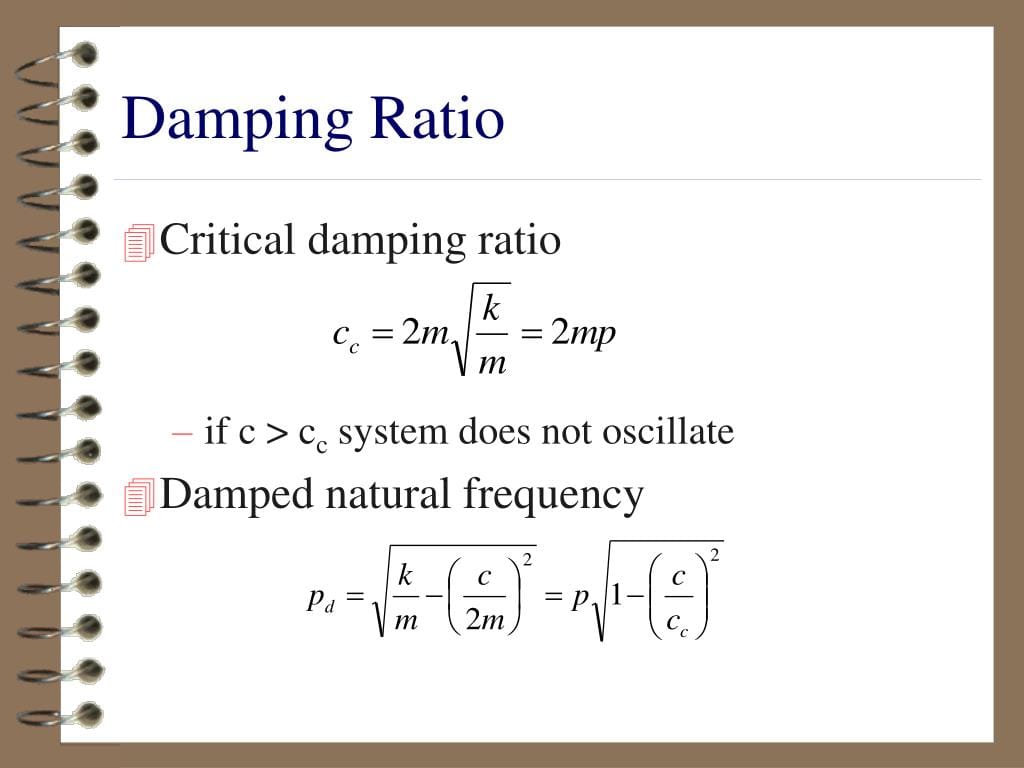
The damping ratio (ζ, zeta) is calculated using the following formula for simple systems:
ζ = c / (2 * √(m * k))
Where:
ζ represents the damping ratio.
c symbolizes the damping constant (resistance to movement).
m stands for mass.
k represents the spring constant (stiffness).
This formula is a ratio of the damping force to the restoring force. Explore the diverse learning opportunities offered by cysd skyward and unlock your child’s academic potential. Consider how damping ratios might be used in designing educational tools and playground equipment.
Real-World Applications of Damping Ratio
Damping ratio isn’t just a theoretical concept. It has tangible real-world applications:
Earthquake-Resistant Buildings: Structural engineers design buildings with specific damping ratios to withstand seismic activity, allowing for safe sway without collapse.
Smooth Car Rides: Automotive engineers tune suspension systems to absorb shocks effectively.
Stable Electrical Circuits: Damping ratios minimize oscillations and noise in circuits.
Precise Control Systems (Robotics and Automation): Damping ensures smooth and accurate movements without overshooting.
Damping Formula: A Deeper Dive
Damping, the gradual energy loss in a vibrating system, is crucial for understanding how things settle down. The damping ratio (ζ = c / cc) measures how quickly oscillations decay. Critical damping (cc = 2√(mk)) is the minimum damping needed to prevent oscillations. The damping equation, m(d²x/dt²) + c(dx/dt) + kx = 0, predicts system behavior over time, considering inertia, damping, and restoring forces.
The Importance of Damping in Various Fields
Buildings and Bridges: Damping systems protect structures from excessive vibrations caused by earthquakes or wind.
Vehicles: Shock absorbers use damping to smooth out bumps and improve handling.
The ideal damping ratio depends on the application. Higher damping ratios offer stability but can be sluggish. Lower damping ratios are responsive but risk instability. Many engineers aim for a damping ratio around 0.7, balancing responsiveness and stability.
Damping Ratio in Different Scenarios
Overdamped (ζ > 1): Slow return to equilibrium without oscillation (e.g., a door closer with too much resistance).
Critically Damped (ζ = 1): Fastest return to equilibrium without oscillation (e.g., a perfectly tuned door closer).
Damping is the gradual loss of energy in a vibrating system, causing oscillations to decay. This energy loss typically transforms into heat due to friction or other resistances. The damping ratio (ζ) quantifies this decay, ranging from 0 (no damping) to values greater than 1 (overdamped).
Types of Damping
Viscous Damping: Resistance from fluids (e.g., oil in a shock absorber).
Coulomb Damping (Dry Friction): Resistance from rubbing surfaces (e.g., a squeaky door hinge).
Hysteretic Damping (Material Damping): Energy dissipation within a material (e.g., a rubber band).
Exploring Further: Ongoing Research and Uncertainties
While much is understood about damping, ongoing research explores more complex systems and phenomena like variable damping. Some experts believe current models may not capture the full complexity of damping in all situations. There’s debate on the most effective calculation methods for highly intricate systems, highlighting the ongoing evolution of this field. This ongoing research suggests that our understanding of damping will likely continue to evolve.
Key Points on Damping Ratio:
Definition: Measures how quickly a system settles after disturbance.
Measurement: Dimensionless number (typically 0 < ζ < 1).
Types of Damping: Undamped, Critically Damped, Overdamped, Underdamped.
Hello, My love for writing is rivaled only by my passion for in-depth research. While many may skim the surface, I dive deep, seeking the hidden gems of information that give a story texture and depth. Every word I write is backed by hours of exploration, ensuring that my work is not just compelling, but also meticulously accurate. Join me on a journey where every piece is a deep dive into the realms of knowledge and storytelling.
