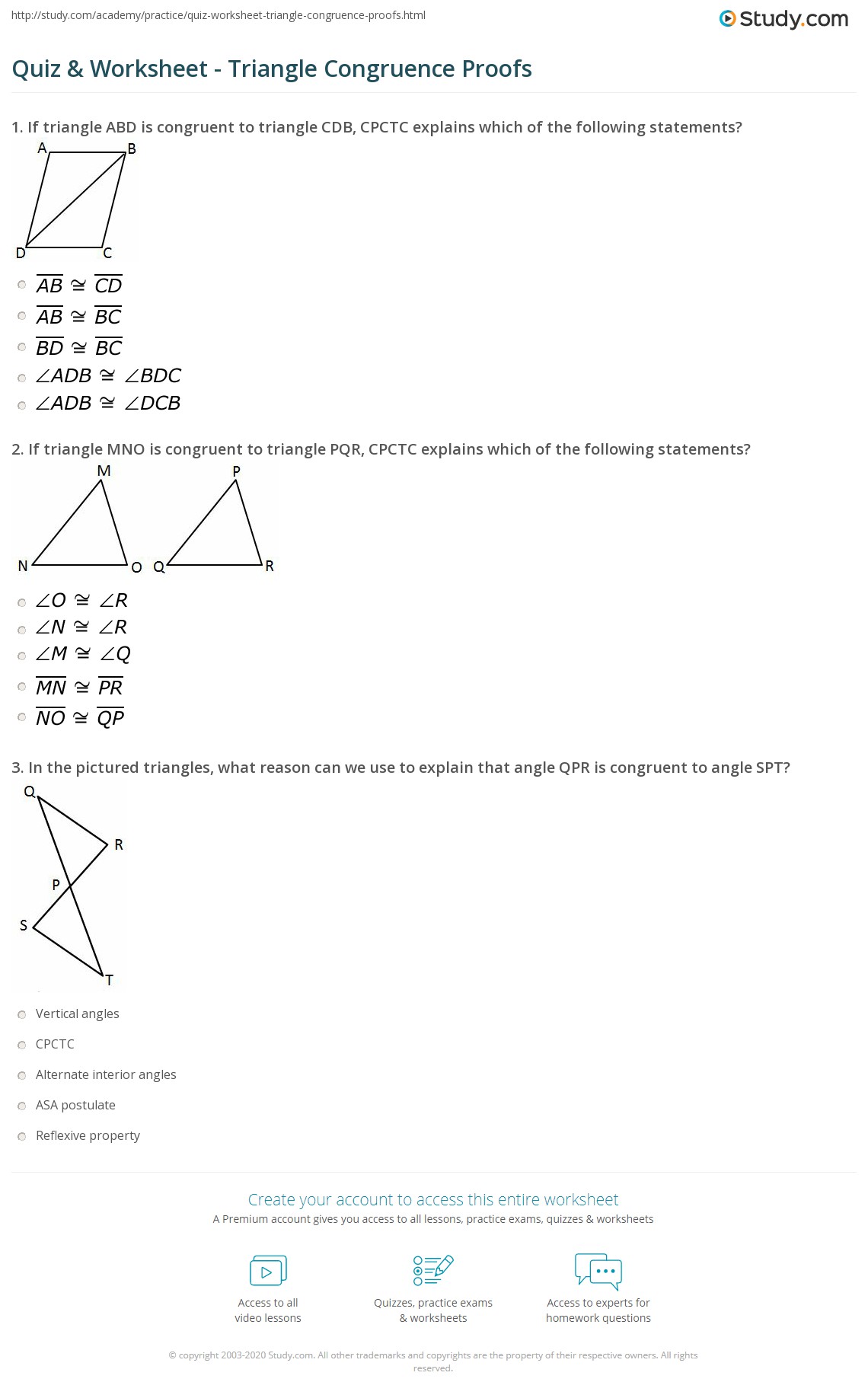
Boost your geometry skills with targeted congruent triangle worksheets! This comprehensive guide provides everything you need to master congruent triangles, from foundational concepts to challenging GCSE-level problems. We’ll explore the key theorems, provide practice exercises, and show you how these concepts apply to the real world. Access a wealth of free printable congruent triangle worksheets with answer keys, catering to all skill levels. Let’s dive in!
Imagine two triangles cut from the same template. They’re identical – the same shape and size. That’s what congruent triangles are! More formally, congruent triangles have corresponding sides of equal length and corresponding angles of equal measure. This seemingly simple concept unlocks powerful problem-solving abilities in geometry. But how do we prove triangles are congruent?
Proving Congruence: Your Geometric Toolkit
We don’t need to measure every side and angle. Instead, we use congruence theorems—our handy shortcuts: SSS, SAS, ASA, AAS, and HL (for right triangles). These theorems are your tools for unlocking geometric mysteries. Let’s explore each one:
SSS (Side-Side-Side)
If all three sides of one triangle are congruent to the corresponding sides of another triangle, then the triangles are congruent. It’s like a triangle’s unique fingerprint.
SAS (Side-Angle-Side)
If two sides and the included angle (the angle between those two sides) of one triangle are congruent to the corresponding parts of another triangle, then the triangles are congruent. The angle acts like a hinge, fixing the triangle’s shape.
ASA (Angle-Side-Angle)
If two angles and the included side (the side between those two angles) of one triangle are congruent to the corresponding parts of another triangle, then the triangles are congruent.
AAS (Angle-Angle-Side)
If two angles and a non-included side of one triangle are congruent to the corresponding parts of another triangle, then the triangles are congruent. This works because if two angles are the same, the third angle must also be the same (since the angles in a triangle always add up to 180°).
HL (Hypotenuse-Leg)
This theorem applies only to right-angled triangles. If the hypotenuse (the longest side) and one leg of one right triangle are congruent to the corresponding parts of another right triangle, then the triangles are congruent.
The Case of SSA (or ASS) and AAA
Why isn’t SSA (or ASS) a valid congruence test? Because two triangles can have two congruent sides and a congruent non-included angle, yet be different shapes. Similarly, AAA proves similarity, not congruence. Triangles with congruent angles can be different sizes.
CPCTC: The Domino Effect
Once you prove congruence, you unlock CPCTC – Corresponding Parts of Congruent Triangles are Congruent. Every corresponding part – every side, angle, altitude, median – is identical.
Congruent Triangles Worksheets: Your Practice Arena
Worksheets are your training ground for mastering congruence. They provide targeted practice for each theorem, building your skills step-by-step. Here are some types of worksheets you might encounter:
Worksheet Type
Benefits
Basic Identification
Practice recognizing congruent triangles based on given information.
Missing Measurements
Use congruence theorems to calculate unknown side lengths or angles.
Proofs
Construct logical arguments to prove triangle congruence using two-column, flow, or paragraph proofs.
Real-World Applications
See how congruence principles apply to practical scenarios.
Congruent triangles aren’t just theoretical; they’re essential in architecture, engineering, and design. Ensuring structural elements are congruent guarantees stability and safety in buildings and bridges. They are also fundamental in surveying, navigation, and even computer graphics.
Tips for Using Worksheets Effectively
Start with easier problems to build confidence, then progress to more challenging exercises.
Always check your answers and learn from your mistakes.
Seek help if you’re stuck—don’t be afraid to ask questions.
Consider exploring interactive geometry tools like GeoGebra.
Beyond the Basics: Further Exploration
While the five congruence theorems are fundamental, ongoing research explores congruence in higher dimensions and non-Euclidean geometries. The field is constantly evolving!
Connecting Concepts: Congruence vs. Similarity
While congruence means identical shape and size, similarity means the same shape, but potentially different sizes. All congruent triangles are similar, but not all similar triangles are congruent. Dive deeper into the composition of substances with this insightful classifying matter worksheet. This helps distinguish between these related but distinct concepts.
By following this guide and utilizing the resources provided, you’ll be well on your way to mastering congruent triangles and achieving GCSE success. Remember, practice makes perfect! So, grab those worksheets and conquer those congruent triangles!
Hello, My love for writing is rivaled only by my passion for in-depth research. While many may skim the surface, I dive deep, seeking the hidden gems of information that give a story texture and depth. Every word I write is backed by hours of exploration, ensuring that my work is not just compelling, but also meticulously accurate. Join me on a journey where every piece is a deep dive into the realms of knowledge and storytelling.
