Ready to conquer the world of trigonometry? This comprehensive guide provides a treasure trove of free printable cheat sheets, interactive tools, expert tips, and memory tricks to help you master sines, cosines, tangents, and more. Whether you’re a beginner or need a refresher, this resource will empower you to tackle trigonometric challenges with confidence.
Essential Trig Resources: Your Fast Track to Mastery
Want to navigate trigonometry with ease? This guide provides an arsenal of resources to help you understand and apply trigonometric concepts effectively.
Quick & Easy Reviews:
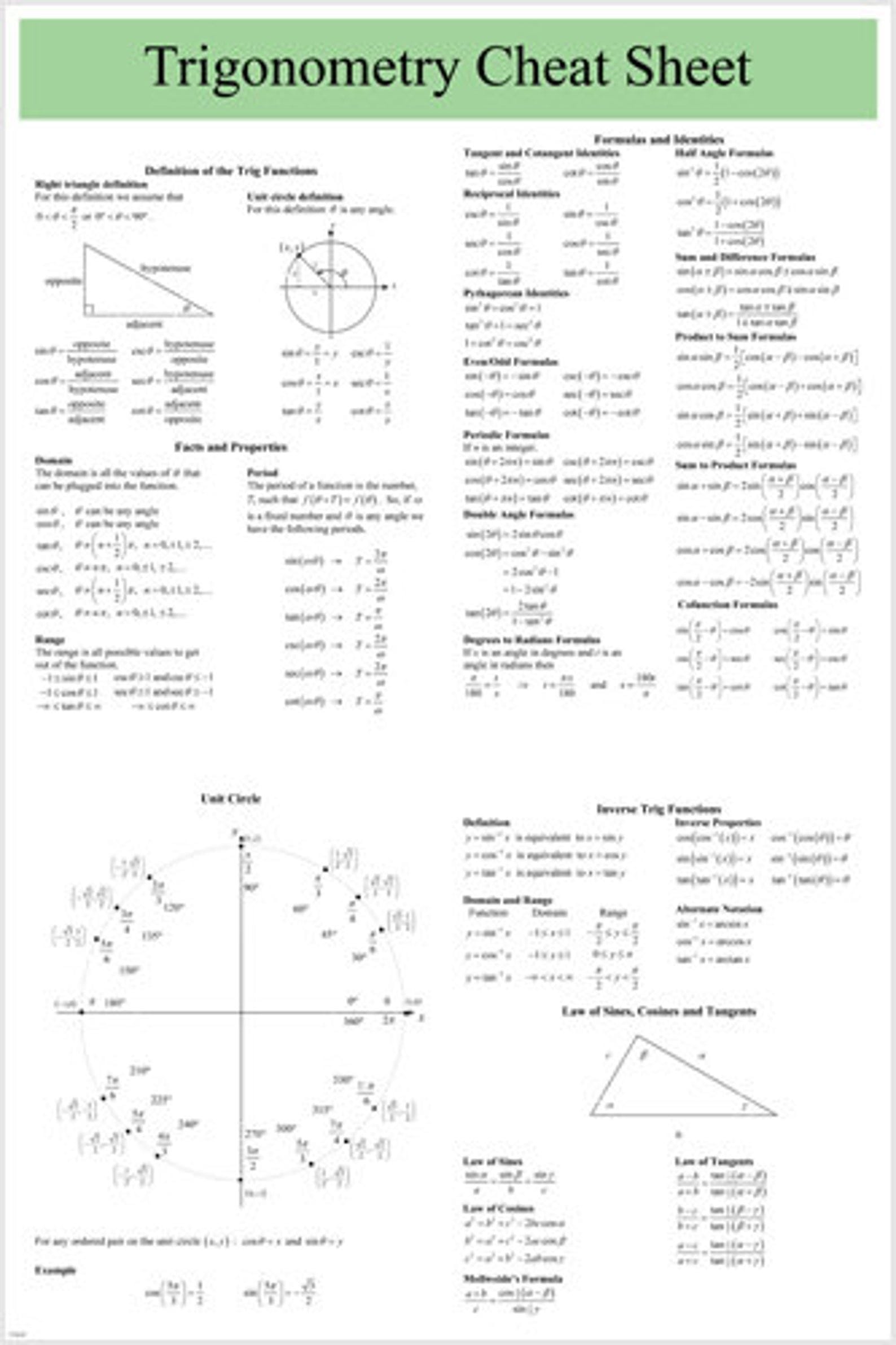
Paul’s Online Math Notes: Need a quick brush-up on the fundamentals? This concise PDF is perfect for a targeted review before a test or when you need a specific formula.
Symbolab: This online cheat sheet offers a comprehensive collection of formulas and concepts, covering both basic and advanced topics.
Deep Dives & Practice:
CompSciLib: More than just a cheat sheet, CompSciLib provides definitions, symbols, formulas, and practice problems with flashcards for enhanced learning and retention. Want to learn more about context clues climber? Here you will find the best strategy that will teach you how to understand the context clues climber.
University of California, Irvine: This resource strengthens your foundation by focusing on defining trigonometric functions and their applications to right triangles.
Advanced Techniques & Applications:
Rapidsheets.net: Take your skills to the next level with this cheat sheet covering advanced equation solving using algebraic techniques and identities. Do you need a cheat sheet trig identities? Click here to see a cheat sheet trig identities that will help you learn the equation.
University of California, Riverside: Bridge the gap between theory and application with this resource, which provides clear definitions and real-world examples of trigonometry in action, particularly with right triangles.
Exam Prep Powerhouses:
Physics & Maths Tutor: Prepare for exams with cheat sheets, worksheets, and model solutions designed to help you ace those tricky questions.
MATHVOX: Deepen your understanding with a dedicated chapter on trigonometric functions, exploring graphs, properties, and laws in detail.
Videos for Visual Learners:
- Trig Substitution Integrals Cheat Sheet: See trigonometry in action within calculus with this video demonstration.
- Trigonometry For Beginners!: Start with the basics using this easy-to-understand video introduction.
- Trig. Identity Cheat Sheet: Visual learners will appreciate this clear overview of key trigonometric identities and their connections.
- A Simple Trick To Remember Trigonometry Values: Unlock helpful memory aids and tricks to recall those crucial trigonometric values.
Beyond the Basics:
Consider these resources for an even richer learning experience:
- Comparison Table: A table comparing the strengths and weaknesses of various cheat sheets will help you find the best fit for your needs.
- Interactive Online Cheat Sheet: Imagine an online cheat sheet with built-in quizzes and practice problems for personalized feedback.
- Specialized Cheat Sheets: Explore cheat sheets tailored to specific fields like engineering or physics.
- The History of Trig: Delve into the fascinating history of trigonometry and the evolution of learning tools.
- Memory Tricks: Discover the power of mnemonics to effortlessly memorize challenging formulas and identities.
Mastering Trig Values: Memory Tricks and Techniques
Memorizing trigonometric values can be challenging. This section explores effective strategies to make those sines, cosines, and tangents stick.
Visual Mnemonics:
- The Hand Trick: This method uses your hand to represent angles (0°, 30°, 45°, 60°, 90°) and calculate sine and cosine values. Detailed explanations and visuals are readily available online.
- Mnemonic Devices: Create or use existing memory rhymes or phrases to associate trig values with their corresponding angles. For example: “Some Old Horses Can Always Hear Their Owners Approaching” (SOH CAH TOA) is commonly used for Sine, Cosine, Tangent values for 0, 30, 45, 60, and 90 degrees.
Understanding Underlying Principles:
- The Unit Circle: This circle with a radius of 1 acts as a map for trigonometric values. Grasping the patterns and relationships between angles and coordinates on the unit circle can greatly simplify value recall.
- Patterns and Relationships: Recognize the inherent symmetry and relationships between trig functions. For example, sine and cosine are complementary functions (sin(x) = cos(90° – x)), and tangent is the ratio of sine to cosine (tan(x) = sin(x)/cos(x)).
- Trigonometric Table: Create a table with angles and corresponding values for each trig function.
Practice and Reinforcement:
- Regular Practice: Consistent practice through quizzes, worksheets, and problem-solving strengthens memory and builds fluency.
- Online Resources: Explore interactive tools, quizzes, and video tutorials for engaging practice opportunities.
| Angle (Degrees) | Sine | Cosine | Tangent |
|---|---|---|---|
| 0 | 0 | 1 | 0 |
| 30 | 1/2 | √3/2 | √3/3 |
| 45 | √2/2 | √2/2 | 1 |
| 60 | √3/2 | 1/2 | √3 |
| 90 | 1 | 0 | Undefined |
While these techniques can be incredibly helpful, true mastery comes from a deeper understanding of the underlying concepts and consistent practice. Experiment with different methods to find what best suits your learning style.
Strategies for Solving Trigonometric Problems
Solving trigonometry problems involves more than just memorization. It requires a strategic approach and understanding of the tools at your disposal.
Key Steps:
- Analyze the Problem: Understand what’s given and what you need to find.
- Choose Your Tools: Select appropriate techniques based on the problem’s structure. Some common techniques include:
- Conjugate Multiplication: Multiplying by a conjugate can simplify expressions, especially those involving fractions with trigonometric functions in the denominator.
- Common Denominators: Essential for adding or subtracting trigonometric fractions.
- Fraction Splitting: Breaking down complex fractions can simplify the problem.
- Rewriting in Terms of Sine and Cosine: This often reveals patterns and simplifies the application of identities.
- Utilizing Trigonometric Identities: Identities are essential for simplifying and solving equations.
- Apply Your Techniques: Carefully execute the chosen techniques, showing all steps.
- Verify Your Solution: Check if your solution makes sense in the context of the problem and satisfies any given equations.
Example: Conjugate Multiplication
Simplifying sin(x) / (1 + cos(x)):
Multiply the numerator and denominator by the conjugate of the denominator (1 – cos(x)):
[sin(x) * (1 - cos(x))] / [(1 + cos(x)) * (1 - cos(x))] = [sin(x) - sin(x)cos(x)] / [1 - cos²(x)]
Using the Pythagorean identity sin²(x) + cos²(x) = 1, we know 1 - cos²(x) = sin²(x):
[sin(x) - sin(x)cos(x)] / sin²(x) = 1/sin(x) - cos(x)/sin(x) = csc(x) - cot(x)
Practice and experimentation are key to mastering these techniques. Research suggests that connecting trigonometry to real-world applications can improve understanding and retention.
Essential Trigonometric Formulas
While there isn’t a definitive list of “7 trigonometric formulas,” this section covers the most crucial and commonly used formulas, along with their applications.
1. Right Triangle Definitions (SOH CAH TOA):
sin(θ) = opposite / hypotenusecos(θ) = adjacent / hypotenusetan(θ) = opposite / adjacent
2. Reciprocal Functions:
csc(θ) = 1 / sin(θ) = hypotenuse / oppositesec(θ) = 1 / cos(θ) = hypotenuse / adjacentcot(θ) = 1 / tan(θ) = adjacent / opposite
| Function | Abbreviation | Relationship |
|---|---|---|
| Sine | sin | Opposite / Hypotenuse |
| Cosine | cos | Adjacent / Hypotenuse |
| Tangent | tan | Opposite / Adjacent |
| Cosecant | csc | Hypotenuse / Opposite |
| Secant | sec | Hypotenuse / Adjacent |
| Cotangent | cot | Adjacent / Opposite |
3. Pythagorean Identities:
sin²θ + cos²θ = 11 + tan²θ = sec²θ1 + cot²θ = csc²θ
4. Angle Sum and Difference Formulas:
These formulas are essential for calculations involving the sum or difference of angles. Their derivations can be found in most trigonometry textbooks.
sin(α ± β) = sinαcosβ ± cosαsinβcos(α ± β) = cosαcosβ ∓ sinαsinβtan(α ± β) = (tanα ± tanβ) / (1 ∓ tanαtanβ)
5. Double Angle Formulas:
sin(2θ) = 2sinθcosθcos(2θ) = cos²θ - sin²θ = 2cos²θ - 1 = 1 - 2sin²θtan(2θ) = (2tanθ) / (1 - tan²θ)
6. Half Angle Formulas:
These formulas are derived from the double-angle formulas.
sin(θ/2) = ±√[(1 - cosθ)/2]cos(θ/2) = ±√[(1 + cosθ)/2]tan(θ/2) = ±√[(1 - cosθ)/(1 + cosθ)] = (1 - cosθ) / sinθ= sinθ / (1 + cosθ)
Beyond these core formulas:
- Sum-to-Product and Product-to-Sum Formulas: Useful for specific types of problems.
- Unit Circle: A visual representation of trigonometric functions and their values for all angles.
- Graphs of Trigonometric Functions: Visualizing sine, cosine, and tangent waves, including amplitude, period, and phase shifts.
- Inverse Trigonometric Functions: (arcsin, arccos, arctan) Used to find angles when given trigonometric values.
This guide provides a solid foundation in trigonometry. Continuous practice and exploration of real-world applications will further enhance your understanding. Remember, the field of mathematics is always evolving, so keep an open mind and embrace the ongoing journey of learning.
- Discover Long Black Pepper: Flavor & Health Benefits - April 25, 2025
- Shocking Twists: The Grownup Review: Unreliable Narration - April 25, 2025
- A Quiet Place Book vs Movie: A Deep Dive - April 25, 2025
















2 thoughts on “The Ultimate Trig Cheat Sheet: Conquer Trigonometry with Free Printable Resources”
Comments are closed.